1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
84
85
86
87
88
89
90
91
92
93
94
95
96
97
98
99
100
101
102
103
104
105
106
107
108
109
110
111
112
113
114
115
116
117
118
119
120
121
122
123
124
125
126
127
128
129
130
131
132
133
134 | % Compare economic vs. tracking mpc.
mpc = import_mpctools();
N = struct('x', 2, 'u', 1, 't', 50);
% Linear model.
A = [0.5, 1; 0, 0.75];
B = [0; 1];
f = mpc.getCasadiFunc(@(x,u) A*x + B*u, [N.x, N.u], {'x', 'u'}, {'F'});
% Objective function.
q = [-2; 2];
r = -10;
lecon = mpc.getCasadiFunc(@(x, u) q'*x + r'*u, [N.x, N.u], {'x', 'u'}, {'lecon'});
Q = [10,0; 0,10];
R = 1;
ltrack = mpc.getCasadiFunc(@(x,u,xs,us) (x - xs)'*Q*(x - xs) + (u - us)'*R*(u - us), ...
[N.x, N.u, N.x, N.u], {'x', 'u', 'xs', 'us'}, {'ltrack'});
% Bounds.
lb = struct('u', -1, 'x', [-10; -10]);
ub = struct('u', 1, 'x', [10; 10]);
% Target finder.
target = mpc.sstarg('l', lecon, 'f', f, 'lb', lb, 'ub', ub, 'N', N, ...
'verbosity', 0);
target.solve();
xs = target.var.x;
us = target.var.u;
ls = target.obj;
% Set up controllers.
par = struct('xs', xs, 'us', us);
econ = mpc.nmpc('l', lecon, 'f', f, 'lb', lb, 'ub', ub, 'N', N, 'xf', xs, ...
'verbosity', 0);
track = mpc.nmpc('l', ltrack, 'f', f, 'lb', lb, 'ub', ub, 'par', par, 'N', N, ...
'xf', xs, 'verbosity', 0);
% Simulate both.
Nsim = 25;
x0 = [-8; 8];
controllers = {econ, track};
names = {'econ', 'track'};
data = struct();
for i = 1:length(controllers)
fprintf('Simulating %s MPC\n', names{i});
x = NaN(N.x, Nsim + 1);
x(:,1) = x0; % Initial condition.
u = NaN(N.u, Nsim);
controller = controllers{i};
for t = 1:Nsim
controller.fixvar('x', 1, x(:,t));
controller.solve();
u(:,t) = controller.var.u(:,1);
x(:,t + 1) = controller.var.x(:,2);
controller.saveguess();
end
data.(names{i}) = struct('x', x, 'u', u);
end
% Get data for objective function contours.
x1 = linspace(-10, 15, 251);
x2 = linspace(0, 10, 101);
[xx1, xx2] = meshgrid(x1, x2);
dx1 = xx1 - track.par.xs(1); % Deviation variables.
dx2 = xx2 - track.par.xs(2);
Vecon = q(1)*dx1 + q(2)*dx2;
Vtrack = Q(1,1)*dx1.^2 + 2*Q(2,1)*dx1.*dx2 + Q(2,2)*dx2.^2;
data.contours = struct('x1', x1, 'x2', x2, 'Vecon', Vecon, 'Vtrack', Vtrack);
% Make plots.
figure();
hold('on');
contour(xx1, xx2, Vecon, 10, '-c');
contour(xx1, xx2, Vtrack, 10, '-m');
colors = {'blue', 'red'};
for i = 1:length(names)
n = names{i};
plot(data.(n).x(1,:), data.(n).x(2,:), '-o', 'color', colors{i});
end
xlabel('x_1');
ylabel('x_2', 'rotation', 0);
% Find rotated cost function.
mu = -(eye(N.x) - A)'\q;
alpha = 0.01;
Mu = dlyap(A', alpha*eye(N.x));
lam = @(x) mu'*(x - xs) + (x - xs)'*Mu*(x - xs);
lrot = @(x, u) q'*(x - xs) + r'*(u - us) + lam(x) - lam(A*x + B*u) ...
- alpha*(x - xs)'*(x - xs);
[x1, x2, u] = meshgrid(linspace(lb.x(1), ub.x(1), 21), ...
linspace(lb.x(2), ub.x(2), 21), ...
linspace(lb.u, ub.u, 21));
l = arrayfun(@(x1, x2, u) lrot([x1; x2], u), x1, x2, u);
fprintf('Minimum of dissipation inequality: %g\n', min(l(:)));
% Simulate multiple economic MPC trajectories.
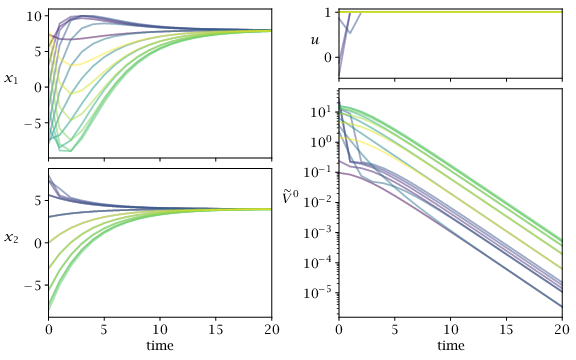
econ.reset();
Nx0 = 16;
colors = jet(Nx0);
theta = linspace(0, 2*pi(), Nx0 + 1);
theta = theta(2:end);
x0 = [8*cos(theta); 8*sin(theta)];
Nsim = 21;
x = NaN(N.x, Nsim, Nx0);
u = NaN(N.u, Nsim, Nx0);
V = NaN(Nsim, Nx0);
plotopts = struct('fig', figure(), 'unames', {{'u', 'V'}}, 'marker', '');
fprintf('Simulating more economic MPC');
for j = 1:Nx0
x(:,1,j) = x0(:,j);
for i = 1:Nsim
econ.fixvar('x', 1, x(:,i,j));
econ.solve();
if ~isequal(econ.status, 'Solve_Succeeded')
warning('%s: failure at time %d!', names{i}, t);
break
end
u(:,i,j) = econ.var.u(:,1);
V(i,j) = econ.obj + lam(x(:,i,j)) - N.t*ls;
if i < Nsim
x(:,i + 1,j) = econ.var.x(:,2);
end
end
fprintf('.');
mpc.mpcplot(x(:,:,j), [u(:,:,j); V(:,j)'], 'color', colors(j,:), ...
'**', plotopts);
end
fprintf('\n');
data.phase = struct('x', x, 'u', u, 'V', V);
|