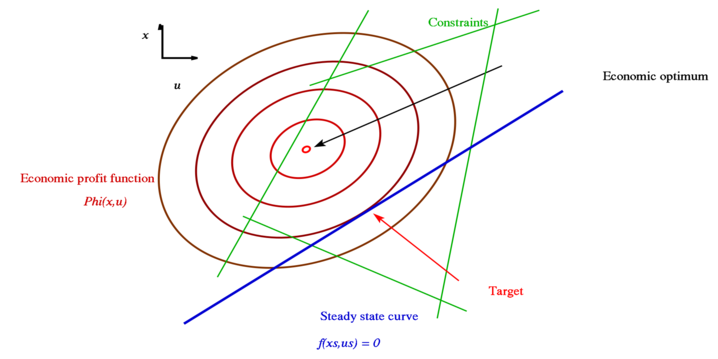
Economic Model Predictive ControlRishi AmritThe overall objective of any process is to convert certain raw material into desired products using available resources. During its operation, the plant must satisfy serveral requirements imposed by its designers and the general technical, economic and social conditions in the presence of ever-changing external influences. Among such requirements are product specifications, operational constraints and safety and enviromnetal regulations. But the most significant incentive for using automated feedback control is the fact, that under the effect of external disturbances, the system must be operated in such a fashion, that it makes the maximum profit. In current practice, this incentive is answered by means of the setpoint/target terminology, which is essentially the process control objective translation of the main economic objective. Translation of objectives in this fashion, results in a loss of economic information and the dynamic regulation layer has no information about the original plant economics except for a fixed steady state target. This work aims at realizing the fact that from a process engineering point of view, the primary purpose of automatic feedback control is not to track setpoints or targets, or to nicely track the dynamic setpoint changes. The primary goal is to operate the plant such that the net return is maximized in the presence of disturbances and uncertainties, exploiting the available measurements. Almost all the literature on automatic control and controller design for chemical processes is concerned with the task to make certain controlled variables track given setpoints or setpoint trajectories while assuming closed loop stability. The work aims at reducing the loss of economic information while solving the dynamic regulation problem, in an attempt to capture higher cumulative profit that is possible with more complete information about the process economics. More detail is presented in the slides from my prelim presentation. |